The conceptual SSC framework described in this section was developed early in the project to provide the entire TI Team with a consistent approach and philosophy to the identification and characterization of seismic sources for use in future PSHAs. The description of the conceptual SSC framework is included in the project report to help the reader understand the basic underpinnings of the SSC model developed for the project, and to show how the framework led to the basic structure and elements of the master logic tree for the SSC model.
The TI Team, which consists of expert evaluators who are responsible for all the technical assessments, includes individuals and organizations with extensive experience in characterizing seismic sources in the CEUS for purposes of PSHA. The TI Team members have experience in PSHAs for nuclear facilities and a variety of other facilities throughout the region. This is a significant benefit to the project in that the team started with a high level of knowledge of the applicable databases for the evaluations, and of the various tools available to assist with the source characterization. Because of this knowledge and experience level, the TI Team was already familiar with the basic tools associated with SSC and uncertainty quantification (e.g., logic trees and probability distributions). So the conceptual SSC framework provided the TI team with the following guidance:
- Reminders of the advanced tools in the “SSC toolbox” for characterizing sources and quantifying uncertainties.
- A systematic approach to use in identifying and documenting applicable data and evaluations of the data relative to its use in SSC.
- Systematic identification and application of various tectonic and seismologic criteria for defining seismic sources that exist within the larger technical community.
The goal was to outline a logical, systematic, and complete framework for characterizing seismic sources within the context of a SSHAC process. To ensure consistency between this framework and the actual SSC effort, the framework was tied directly to the master logic tree of the SSC model.
Organized in this section are the concepts developed over the years for assessing seismic sources within stable continental regions (SCRs), including the CEUS. An early version of this section provided a useful tool to the team members during the course of their evaluations and to the peer reviewers, who sought to understand the framework within which the team worked. After the actual SSC effort was completed, this section was refined to reflect the actual project implementation and it became a part of the project report documentation.
4.1 Needs for a Conceptual SSC Framework
In consideration of the purpose of the CEUS-SSC Project, the TI Team identified three attributes that are needed for a conceptual SSC framework:
- A systematic and documented approach to treating alternatives using logic trees, including alternative conceptual models for future spatial distributions of seismicity (e.g., stationarity), alternative methods for expressing the future temporal distribution of seismicity (e.g., renewal models, Poisson models), and alternative data sets for characterizing seismic sources (e.g., paleoseismic data, historical seismicity data).
- A systematic and documented approach to identifying applicable data for the source characterization, evaluating the usefulness of the data, and documenting the consideration given to the data by the TI Team.
- A methodology for identifying seismic sources that is based on defensible criteria for defining a seismic source, incorporates the lessons learned in SSC over the past two decades, and identifies the range of approaches and models that can be shown to be significant to hazard.
The need for an SSC framework that would fulfill these needs was encouraged by the PPRP early in the project, and the PPRP provided valuable feedback during the course of developing the framework. Each of these needs has been addressed in the development of the framework for the project, as discussed in Sections 4.1.1 through 4.1.3 below.
4.1.1 Logic Tree Approach to Representing Alternatives and Assessing Uncertainties
Over the past 25 years, it has become clear that a significant contribution to epistemic uncertainties in SSC comes from uncertainty in alternative conceptual models. Logic trees provide an effective means of clearly representing the credible alternative models and assigning weights to the alternatives. Logic trees were originally defined in a probabilistic framework for use in PSHA (e.g., Kulkarni et al., 1984) with a specification that the values on the branches of the tree be mutually exclusive and that all branches at a node of the tree be collectively exhaustive. Some have called this assumption into question in common applications, because it is often not possible to prove that all branches have been included or that they are completely mutually exclusive (Bommer and Scherbaum, 2008). Nevertheless, with these cautions in mind, logic trees provide a practical means of representing alternative hypotheses, expressing the relative weight for each hypothesis given the available data, and combining the hypotheses for use in the hazard analysis.
Logic trees have become common tools for application in SSC and specifically for the model-building or integration phase of a SSHAC project (Section 2.1), and the TI Team used them for expressing the epistemic uncertainties in alternative methods and approaches to characterizing sources. For example, the first elements of the master logic tree (discussed in Section 4.2) define the basic alternative approaches to defining seismic sources as a function of the criteria used. Once these approaches are defined and the relative weight for each is assigned, the subsequent characterization will follow the approach defined for that branch. Thus the “logic” that comes into play in a logic tree is defining the dependencies among the assessments on the branches, and the outcomes that derive from each branch. Therefore, logic trees typically begin with general assessments (e.g., alternative conceptual models) and proceed to more specific assessments that are conditional on the general assessments. The assessments found to the right on the logic tree are commonly the specific elements and parameter values that are associated with a particular conceptual model. For example, an assessment of alternative temporal models (e.g., Poisson versus renewal) would be to the left on the logic tree, and each model would then be defined by nodes to the right that define the required parameters for each model (e.g., mean recurrence, elapsed time, coefficient of variation of recurrence intervals).
4.1.1.1 Examples of Logic Trees
Examples of logic trees used in actual projects are shown on Figures 4.1.1-1 and 4.1.1-2. The first figure comes from the PEGASOS project in Switzerland (NAGRA, 2004). The SSC team evaluated the potential that Permo-Carboniferous troughs within the Molasse basin of Switzerland might be seismogenic and localizers of moderate to large earthquakes. The first node of the logic tree identifies this evaluation and the relative probabilities assigned to the alternative hypotheses (reactivated or inactive). A second example logic tree is given on Figure 4.1.1-2, which comes from the probabilistic volcanic hazard analysis for Yucca Mountain (SNL, 2008). This tree begins with the assessment of the relevant volcanic events to be considered for the analysis, then proceeds to the alternative spatial and temporal models identified to define the future distribution of volcanic events. In this case, the weights assigned to the alternative branches are expressed as percentages, with the branches at a particular node summing to 100 percent. Regardless of the form-probabilities or percentages-the values on the branches are weights that represent an assessment of the relative credibility of the alternatives given on each branch.
4.1.1.2 Assigning Weights to Logic Tree Branches
In some cases, continuous parameter distributions can be accurately defined by a discrete set of logic tree branches and associated weights. However, in most cases in the CEUS-SSC Project, the weights assigned to the branches are subjective and based on the TI Team’s assessment of the relative support for the alternative branches, given the available data. Although the final assignments of weights to logic tree branches are subjective, the weights represent assessments informed by the totality of the SSHAC evaluation process. Before weights were assigned, the TI Team heard from a properly wide range of resource and proponent experts, reviewed extensive technical information, created the Data Summary and Data Evaluation tables, and evaluated a wide range of issues with members of the knowledgeable broader technical community. In this way, the subjective weights are informed by the consideration of data, models, and methods in the evaluation phase of the SSHAC process.
Across all assessments in the SSC model, the total set of logic tree branches and weights represent the team’s assessment of the center, body, and range of technically defensible interpretations (see Section 2.1 for a discussion of this concept). Those assessed alternatives that are judged not to be credible should not be included in a logic tree. In some cases, it was deemed helpful in the project documentation to identify those alternatives that have not been included, and the basis for not including them, but there is no requirement to include the global set of noncredible alternatives. An example of a noncredible alternative might be a model that has been proposed in the literature but whose application to a particular seismic source would violate the available data. A recurrence model that would over predict the observed seismicity by orders of magnitude after accounting for uncertainties in catalog completeness is an example of an alternative that can be assigned zero weight and not be included in the logic tree. A discussion in the report that such a model was considered and rejected assists the reader in understanding the full range of considerations made by the evaluation team.
The weights applied to the branches of the logic trees reflect the TI Team’s assessment that the particular branch is the correct branch. It is important to note that the TI Team spent considerable effort identifying alternative logic tree branches to be included that are significantly different from one another-from a hazard point of view. For example, at an early point in the project, alternative source geometries were postulated for the Charlevoix zone. However, the differences between the alternatives were minor for use in a regional seismic hazard model and so did not warrant incorporation into the source logic tree as two separate branches. Commonly, for purposes of PSHA, the branches are used to represent data, models, and methods that have some level of credibility as the correct parameter value, model, or method. It is the available data and information that provide the basis for the TI Team’s assessment of the relative weights. If there is no basis in the available data for a preference from among the alternative branches, then the weights will be the same for all alternatives. For example, if the available data give equal support to two alternative positions of a seismotectonic zone boundary, then the alternatives are assessed equal weight.
For purposes of illustration, assuming there are two alternative branches in a logic tree, a higher weight is assessed for one of the alternatives if there is a technical basis in the available data to do so. Moving from weights of 0.6/0.4 (slight preference) to 0.9/0.1 (strong preference), the relative preference for the alternatives is becoming more pronounced, reflecting the stronger technical support for one of the alternatives. Although numbers (weights) are being used, these are treated as subjective probabilities and there is rarely a quantitative basis for assigning these weights. Exceptions on the CEUS-SSC Project are the five-point distributions to represent quantified continuous distributions of selected parameters (for example, see the description of recurrence parameters for RLME sources in Section 5.3.3.1.3). The TI Team evaluated the alternatives using available data and information and made its best attempt to represent the present uncertainty. The Team reviewed the positions of various proponents of the alternatives, if those positions have been taken, and considered the degree of support the alternatives would have if members of the technical community were aware of all the project databases and had gone through the interactive SSHAC process of evaluating the alternatives. Ultimately, the key to the use of logic trees is clear documentation of the models/parameters that are given on the branches of the tree and justification for the weights assessed for the various branches. For example, the seismic source characteristics for the seismotectonic zones are given in Section 7, along with a discussion of the technical bases for all assessments and weights on the logic tree.
4.1.2 Data Identification and Evaluation
Documentation of SSC requires that the data be identified that were considered and used in the analyses. The term “data” is used in a general sense to indicate all types of information that have potential use in defining and characterizing seismic sources for PSHA. By identifying the data, the reader will understand the technical bases for the assessments and, if some time has passed since the project was conducted, will have information about the data and references that were available and considered at the time of the project. Also, the documentation should preferably include an assessment of the quality of the data and the degree of reliance that was placed on various data sets.
Data identification and evaluation occur at the earliest part of a PSHA project and continue until the model-building or integration process is complete. A distinction is made between data identification, which is the process of becoming aware of and compiling available information having relevance to SSC, and data evaluation, which is the process of assessing the quality and applicability of the information to SSC. The process by which the data were identified and evaluated for the CEUS-SSC Project is discussed in Sections 4.1.2.1 and 4.1.2.2.
4.1.2.1 “Generic” Data Identification to Address Indicators of a Seismic Source
For purposes of the CEUS-SSC Project, the data identification process was informed by available guidance issued for this purpose, as well as by the experience of members of the TI Team in conducting SSC projects. Existing guidance documents provide recommendations as to the types of data that can be useful in defining seismic sources. For example, Table 4.1.2-1 is taken from the standard ANSI/ANS-2.27-2008, Criteria for Investigations of Nuclear Facility Sites for Seismic Hazard Assessments, and indicates the types of data that can be used to identify and characterize fault sources and areal source zones (American Nuclear Society, 2008a). Table 4.1.2-2 provides another example taken from the SSHAC guidance (Budnitz et al., 1997). It includes a further specification of data that can be used for various source types, as well as an evaluation of the relative usefulness of various types of data for identifying and characterizing seismic sources. These types of summaries are useful at the outset of an SSC project in focusing the database identification and compilation efforts toward the data that are likely to be useful in characterizing seismic sources for PSHA.
For the CEUS-SSC Project, the data identification process is “generic” in the sense that it applies to the entire CEUS study region and not to any particular seismic source or subregion. Rather than tie the data to particular types of sources (e.g., faults, source zones), the types of data are identified that can be used to address a variety of “indicators” of a unique seismic source (Table 4.1.2-3). Table 4.1.2-3 documents the evaluation of the indicators of seismic sources and the relative usefulness of various types of data that can be used to address the indicators. This assessment is similar to that given in the examples in Tables 4.1.2-1 and 4.1.2-2, but provides further evaluation of possible indicators of seismic sources and of the relative usefulness of various data in addressing those indicators.
The assessment of possible indicators of seismic sources and their relative value is necessarily subjective and reflects the TI Team’s consideration of the current views of the SSC community. Also, the indicators are particularly pertinent to the CEUS, which is an SCR (Johnston et al., 1994) in which the causative faults giving rise to seismicity are generally not known. Therefore, unlike an assessment of indicators of seismic sources in an active plate boundary region, the indicators within the CEUS are more uncertain and vary from evidence of geologically young deformation, to observed zones of earthquakes, to other types of geologic and geophysical evidence. The types of data that are potentially useful in addressing these indicators also vary. The generic data identification in Table 4.1.2-3 is intended to associate the types of data that may be useful for SSC with potential indicators of seismic sources. In this way, as the knowledge of the technical community evolves regarding the most important indicators of seismic sources in the CEUS, the table can be updated to reflect that evolution. Also, if particular data types emerge in the technical community as being more diagnostic in defining seismic sources, those data types can be assigned higher weight in the table.
The various columns of Table 4.1.2-3 are defined and discussed below.
- The first column is a listing of possible indicators of a unique seismic source. If we assume that we start with a map of the entire CEUS, these are the indicators that could cause one to consider subdividing the region spatially to indicate a unique potential seismic source. Further, it is assumed for purposes of this table, which is a generic evaluation, that the indicator is known with certainty. In application to any particular region, there may be uncertainty as to whether the indicator exists.
- The second column is an evaluation by the TI Team of the relative usefulness of each of the indicators in identifying seismic sources. Note that the indicators and evaluation of their usefulness are snapshots of the knowledge at the time this table was made. It is expected that future scientific studies will provide additional insights into the causative factors related to CEUS seismicity. Accordingly, the relative usefulness of various indicators can be expected to change with time.
- The third column is a listing of the types of data that can be used to address the indicators. This list builds on previous efforts to identify the types of data that are potentially useful for characterizing seismic sources, including those shown in Tables 4.1.2-1 and 4.1.2-2.
- The fourth column provides an evaluation of the relative usefulness of each data type in addressing the indicators. Because the evaluation of usefulness is a function of both data type and quality of the data, it is assumed for this assessment that high-quality data are available. For example, consider the indicator “high strain rates.” This indicator is assigned a relatively high level of usefulness (a score of 4) for identifying a seismic source. Two types of data are identified for addressing this indicator: (1) tectonic geodetic strain data and (2) geologic indicators of recent strain. In evaluating the relative usefulness of the two data types, it should be assumed that good-quality geodetic data as well as geologic data are available. Given this assumption, the geodetic data are assigned a moderately high usefulness (score of 4), and the recent geologic data are assigned a higher usefulness (score of 5) in addressing the high-strain-rate indicator. This is because the geologic data would span a longer period than the geodetic strain indicators.
- The fifth column is an identification of the part of the SSC model that would be affected by the indicator. The aspects of the model are the spatial component, which describes the location and geometry of seismic sources, or the temporal component, which describes the recurrence rate and magnitude distribution. One or the other or both components may be affected.
The assessments given in Table 4.1.2-3 provided a basis for the TI Team to identify the applicable data that should be compiled for purposes of SSC. The weights assigned to potential indicators of seismic sources and to the usefulness of various data types were not used in a quantitative way in the project. Rather, they provide a basis for documenting the current thinking regarding the relative importance of potential indicators and the relative usefulness of various types of data to address the indicators. They also provided a means of prioritizing the data compilation efforts toward those data that have the highest potential usefulness in the SSC process. For example, paleoseismic indicators of M > 5 earthquakes are judged to be highly diagnostic indicators of seismic sources, whereas zones of weakness in the crust or mantle are given a relatively low weight as an indicator. Spatially concentrated earthquakes are given a high weight. Consistent with these assessments, the TI Team turned the focus of the project database toward the development of a new earthquake catalog and devoted a major effort to compiling paleoliquefaction data.
4.1.2.2 Data Evaluation for Particular Seismic Sources: Data Evaluation and Data Summary Tables
The second part of the data identification and evaluation process is the identification of specific data that were considered and used to characterize particular seismic sources, including RLME sources or seismotectonic zones. The purpose of this evaluation is to identify the data used, evaluate the quality of the data, and specify the degree of reliance on each data set in characterizing seismic sources. Data Evaluation tables were developed for this purpose (Table 4.1.2-4 is an example), and the tables for each source are included in Appendix C. The process also provides an opportunity to identify data sets that were considered in the evaluation even if they were not ultimately used to characterize seismic sources. Data Summary tables were developed for this purpose (Table 4.1.2-5 is an example), and those tables are included in Appendix D.
The Data Evaluation tables include the following attributes (see Table 4.1.2-4):
- The first column is a listing of the data, by data type, used in the evaluation for a particular RLME or seismotectonic source.
- The second column is an assessment of the quality of the data by the TI Team. This assessment is qualitative and takes into account the resolution, completeness, and distribution of the data relative to the best data of that type currently available. In some cases the assessment of the quality of a particular data set differs somewhat for different seismic sources. This is a reflection of the perceived value of the particular data set toward addressing the SSC characteristics of each seismic source.
- The third column is used for notes about the data quality. This usually includes comments about whether the data have been published in abstract form or full papers and other issues regarding the defensibility of the data.
- The fourth column identifies the particular seismic source to which the data have been applied in the evaluation.
- The fifth and sixth columns provide an assessment of the degree of reliance on the data set for purposes of SSC, and a short description of how the data were relied on. The intent is to assist the reader in understanding how the data set was used and what the evaluation of the degree of reliance was based on.
- The seventh column indicates whether the data exists in GIS format within the project database. If the data are not in GIS format, they will be found in the database in other formats such as a PDF file.
Data Summary tables (example in Table 4.1.2-5) provide information on the various data that were considered during the course of the characterization of various seismic sources. The tables provide the citations to the data and a description of the key conclusions and their potential relevance to SSC. The goal is to provide the reader with the TI Team’s view of the data set and how it might pertain to SSC. This can be particularly useful to other researchers-perhaps some years from now-in understanding what data sources were considered at the time of the CEUS-SSC and how their relevance was assessed.
The Data Evaluation and Data Summary tables are not intended to replace the documentation of an SSC effort, but rather to supplement it. The discussions in a project report of the data used in the evaluation are not always comprehensive and it can be difficult to gain a clear understanding of exactly which data sets were considered, which were actually used in the evaluation, and the degree of reliance that was placed on them. Therefore, these tables were designed to make the data evaluation process more transparent and reasonably complete. It should be noted that these tables in particular-and the documentation in general-are a snapshot of a particular point in time. That is, the types of data available and their quality and utility are a function of our present understanding of SSC for PSHA. It is likely that in the future, additional data will become available that will prove useful for identifying or characterizing seismic sources.
Also, it is likely that the degree of reliance on any given data set will change in the future. For example, at the present time, GPS geodetic data are available for only a relatively limited part of the CEUS, and the period of observation is relatively short, such that errors in the data may exceed the signal. Moreover, it is not clear, given our present understanding of earthquake strain accumulation processes, exactly how geodetic strain rates provide direct constraints on seismic source characteristics. For example, the Independent Expert Panel on New Madrid Seismic Zone Earthquake Hazards (NEPEC, 2011) concluded that the observations of a lack of deformation based on the geodetic evidence were not sufficient to rule out the potential for future large earthquakes. Yet it is likely that uncertainties in the use of geodetic data will decrease with time and that this data set will become more valuable in the future for SSC. It is also possible that entirely new types of data will become available in the future on which the SSC community will become increasingly reliant.
Although the CEUS-SSC Project placed a premium on compiling a wide variety of databases and placing many of the databases on a common GIS platform to facilitate their use, not all of the data were used directly in the characterization of seismic sources. This is simply because some data and references have been superseded by later studies or some types of data are viewed as having only a limited usefulness in meeting the criteria for defining seismic sources. Nevertheless, the documentation process followed in the data tables will allow the reader to understand which data were considered in the course of the evaluation process, as well as which data were relied on in the seismic source model.
4.1.3 Methodology for Identifying Seismic Sources
The methodology used in the CEUS-SSC Project to identify seismic sources takes advantage of the experience gained over the past three decades in assessing SSCs for PSHAs. It incorporates the range of views in the scientific community regarding spatial stationarity of seismicity, and it is appropriate for a regional SSC assessment that can be applied on a consistent basis throughout the CEUS. A regional PSHA requires that the assessment include elements that are of sufficient specificity to include new thinking and contemporary data on seismic sources, yet is not reliant on site-specific information that cannot be applied systematically throughout the entire CEUS. Further, over time, new data are likely to be developed on a site-specific basis, thus calling for a stable regional model that can be refined for future new findings. For example, in recent years, paleoliquefaction data have been gathered and interpreted at particular locations, such as the New Madrid, Charleston, and Charlevoix seismic zones. For the vast majority of the CEUS, however, such features may not be present or data may not have been systematically gathered and evaluated; thus they are not available for incorporation into a hazard analysis. Accordingly, the SSC methodology advanced in this project can allow for the incorporation of such data in those cases where it is available, but given the incomplete distribution of the data across the region, the methodology should not assume or require that such data be available throughout the regional SSC model.
Workshop #2 on Alternative Interpretations provided an opportunity for the TI Team to discuss with members of the technical community several important issues with potential relevance to the identification of seismic sources. For example, paleoseismic indicators of possible RLME sources were discussed, including locations with strong evidence and those with equivocal evidence. In particular, considerable discussion in the workshop centered on the evidence for the location, size, and timing of earthquakes based on paleoliquefaction evidence. Given the potentially high significance of these types of data and their increasing credibility within the technical community as indicators of seismic sources, the project and the TI Team were encouraged by the PPRP to place high priority on the identification and evaluation of paleoliquefaction data and to complete the paleoliquefaction database that culminated in Appendix E to this report. Another issue discussed was the degree to which the spatial patterns of observed earthquakes provide an indication of future patterns. Proponents dealt with the issue of observed geodetic strain rates and their consistency with the presence or absence of sources of large earthquakes identified by other means. Likewise, alternative possible tectonic explanations were proposed to explain concentrations of observed seismicity, with the potential implications of using those explanations to define seismic sources. All of these issues have potential implications for defining the criteria for source identification in a meaningful way. That is, the criteria must take into account the technical community’s views of the important indicators of seismic sources and they must also be implementable across the study region given the available data.
It is assumed that the methodology outlined in this section will provide the regional component of the SSC, which is subject to refinement with the consideration of site-specific data and information. For example, the output from this project will be a reasonably complete specification of the knowledge and uncertainty regarding the spatial and temporal aspects of seismic sources on a consistent basis throughout the CEUS study region. It can therefore be exercised in a PSHA (which will include ground motion characterization) at any location in the study region. If the results are to be used for purposes of licensing at a particular nuclear facility location, regulatory guidance (e.g., NRC Regulatory Guide 1.208) requires that a site-specific database be developed. Similar guidance for other nuclear facilities requires the consideration of local and site-specific information (e.g., ANSI/ANS-2.27-2008, ANSI/ANS-2.29-2008). Once it is developed, the applicant will need to evaluate whether the site-specific database includes information pertinent to SSC and, if it does, then the site-specific information will need to be incorporated into the CEUS-SSC source model. Alternatively, the applicant might consider the hazard significance of the site-specific information and determine that it would not require a refinement to the CEUS-SSC model (see Section 9.4.3 for a discussion of hazard significance).
The concept of a “regional” SSC model is easily understood, as is the type of “site-specific” information that is commonly developed to support a regulatory license application under current regulatory guidance. However, the TI Team has considered whether further specification can be made of what is considered “regional” and what is considered “site-specific.” In other words, is there a “scale cutoff” below which one would consider the data too local to be systematically characterized throughout the entire study region? Clearly, local tectonic features that lie entirely within the 8 km (5 mi.) radius site area, and likely the 40 km (25 mi.) radius site vicinity, as defined in NRC Regulatory Guide 1.208, would be too site-specific to be included on a systematic basis in the CEUS-SSC source model. Unless special studies have been carried out that demonstrate the existence of tectonic features having a significant seismogenic potential, the consideration and potential incorporation of specific tectonic features would be part of the refinement of the CEUS-SSC model for site-specific application. Thus the TI team is unable to specify a quantitative cutoff dividing regional from site-specific.
A more reasonable criterion that was applied in the CEUS-SSC Project is the following: the CEUS-SSC model provides the regional characterization of sources on a consistent basis throughout the study region, including those special areas that have been the subject of considerable scrutiny in the past. Consideration of site-specific refinement of the CEUS-SSC model would be required by current regulatory guidance and would occur only if such refinement would lead to significant differences in hazard.
4.1.3.1 Hazard-Informed Approach
Numerous PSHAs have been conducted within the CEUS and other SCRs over the past three decades. The experience gained over that time was used in defining the framework for identifying and characterizing seismic sources. The knowledge gained on the important SSC issues will likely contribute to the hazard results at annual frequencies of exceedance important to nuclear facilities. Likewise, the most important contributors to uncertainty can be anticipated. It is also possible to anticipate those technical issues that will have lesser or no significance to the hazard results.
For example, SSC studies conducted in the 1980s, such as the EPRI-SOG project, focused on the issue of evaluating the probability for particular tectonic features to localize moderate to large (M ≥ 5) earthquakes within the contemporary tectonic regime. The evaluation of this probability of activity, Pa, was viewed as a fundamental part of the SSC process. Included in the evaluation were hypotheses related to the causative mechanisms of CEUS seismicity, the nature of the contemporary stress regime, and various data indicators that would provide insights into whether a tectonic feature-or class of features-might be seismogenic. These 1980s assessments provided valuable insights into the then-current state of knowledge and uncertainty about the causes of CEUS earthquakes. We can take advantage of these insights in outlining our SSC approach some 25 years later.
One of the insights gained from experience on several PSHAs is that observed seismicity is perceived by the larger technical community as providing a fundamental constraint on estimates of the future spatial and temporal distribution of moderate to large earthquakes. This is despite the heavy emphasis placed on studies like the EPRI-SOG project on tectonic features and their potential to be seismogenic. Examples of the reliance on observed seismicity in these studies can be found in several source types. Within the more active zones, such as New Madrid, the seismicity data were used to define the spatial location of the seismic sources as well as the recurrence rates for the sources. Away from the more active zones, background zones were identified whose probability of activity was typically 1.0 and whose recurrence rate was defined by the diffuse seismicity within the zone. Seismicity within large background seismic source zones was also used to “smooth” recurrence parameters (a- and b-values), providing for spatial variations based on seismicity.
The assessment of maximum earthquake magnitudes for seismic sources within the CEUS is typically not constrained by physical characteristics of the source itself (e.g., fault rupture length); instead, it is estimated considering the largest earthquakes within the seismic source as well as analogues to other sources that are tectonically similar. Even in those cases where tectonic features were identified as candidates for localizing future M ≥ 5 earthquakes, the most diagnostic criterion for evaluating seismogenic potential was the spatial association of the feature with observed seismicity. Those tectonic features that were assessed to have a low probability of being spatially associated with seismicity (often due to low numbers of observed earthquakes) were assigned a low probability of being seismogenic, Pa, regardless of any existing evidence. In nearly all cases, conclusive geologic evidence for recent fault displacement-which would be a diagnostic criterion if it did exist-simply was not identified in the available data. As a result, in nearly all cases, the hazard significance of individual tectonic features was assessed to be very low to negligible.
We conclude from this experience that the characteristics of the observed seismicity record- both the spatial and temporal distribution of earthquakes-are important constraints and have high hazard significance. Therefore, the SSC methodology advanced in the CEUS-SSC Project appropriately places heavy emphasis on the systematic and consistent development of seismicity databases and on approaches to their use in defining and characterizing seismic sources. Conversely, less emphasis is placed on identifying and evaluating tectonic features that are not clearly associated with observed seismicity or that do not show geologic evidence of recent activity within the present tectonic regime.
This should not be interpreted as suggesting that the earthquake community has discarded the search for associations between earthquakes and tectonic features within SCRs, or that observed seismicity provides an unequivocal description of future earthquakes. Earthquake research within SCRs continues to hypothesize a variety of possible mechanisms for the observed seismicity; spatial associations with deep crustal or mantle anomalies are such candidates. Rather, it reminds us that the purpose of the CEUS-SSC Project is to develop a seismic source model to be used in a seismic hazard analysis, and not to answer research questions about SCR earthquake causative mechanisms. Postulated spatially and/or temporally clustered/episodic behavior of large-magnitude earthquakes at New Madrid is an example of a hypothesis that has potentially significant hazard implications and that is addressed directly in the seismic source model.
An additional insight gained during the past 20 years, due largely to a number of geologic studies conducted over that period, is that paleoseismicity is important and its potential for hazard assessment is very significant. Beyond the observed historical and instrumental seismicity record, no single data set has had a more profound influence on matters of maximum size of SCR earthquakes, their spatial distribution over periods much longer than the historical record, and the rates and behavior of currently active seismic sources. With very few notable exceptions, such as the Meers fault, the paleoseismicity evidence has been based entirely on shaking effects rather than observed displacements along the causative fault. For this reason, the causative structures giving rise to the paleoearthquakes remain elusive in most cases. Likewise, significant uncertainties exist regarding the locations, magnitudes, and recurrence of the earthquakes based on the geologic record. Nevertheless, the existence of the paleoearthquakes is in most cases undeniable and, because of their potentially high hazard significance and the technical community’s general support, they must be incorporated explicitly into the seismic source model. Because the causative faults for these earthquakes are not known, the paleoearthquakes can be viewed as simply an extension of the observed seismicity catalog back in time. Of course, in doing so, care must be taken to properly evaluate the interpretation of paleoseismic evidence and assess the uncertainties in the size and timing of the earthquakes.
To further identify and understand the issues of most hazard significance, seismic hazard calculations were conducted using the SSC sensitivity model prior to Workshop #3 for a series of sensitivity cases. The issues identified as having the most hazard significance were as follows:
- Large-magnitude sources (e.g., New Madrid, Charleston, Charlevoix)
- Magnitude of the “characteristic” (repeated large-magnitude) earthquake
- Recurrence rate
- Location of the source
- Moderate-magnitude sources (e.g., Eastern Tennessee seismic zone, Central Virginia seismic zone, Wabash Valley)
- Source geometries
- Maximum earthquake magnitude
- Recurrence rate
- Smoothing (i.e., whether seismicity is distributed uniformly within the zone or smoothed locally)
- Background zones
- Maximum earthquake magnitude
- Smoothing
- Probability of activity (i.e., whether the zone has a Pa less than 1.0)
These findings reinforce the importance of focusing on the locales that have hosted moderate- to large-magnitude earthquakes in the observed seismic record, and of using that record, along with other indicators such as paleoseismic information, to define the location/geometry, maximum size, and recurrence rates. Away from those locales, issues related to the seismogenic potential of the background regions were found to be important if the Pa was judged to be less than 1.0; that is, if there was some finite probability that the region was not capable of generating a M ≥ 5 earthquake. However, with time and continued study of SCRs around the world, there is increasing consensus that any region within an SCR is capable of generating earthquakes of those magnitudes. Further, the uncertainties in this assessment can be readily addressed in the assessment of Mmax for the zones. Therefore, the Pa issue for background zones has much less hazard significance.
4.1.3.2 Conclusions Regarding the Hazard Significance of Various SSC Issues
Based on the experience of multiple PSHAs in the CEUS since the time that major studies were conducted in the 1980s, as well as sensitivity studies conducted for the CEUS-SSC Project, the following conclusions can be drawn regarding the most important SSC issues and their implications in developing an SSC methodology.
- Despite continued study, the causative structures (faults) for the observed moderate- to large-magnitude earthquakes in the CEUS remain unknown, with very few exceptions. Thus a seismic source model comparable to those developed in the WUS (e.g., faults with background zones) is not possible.
- The observed record of seismicity, despite uncertainties in the locations and magnitudes of earthquakes, and the completeness of the record, is the fundamental means of assessing the future locations, sizes, and rates of earthquakes needed for a PSHA. Our tools for quantifying the uncertainties in the record have become better developed, as have our tools for using the
record (e.g., spatial smoothing).
- Evaluations of potential causative tectonic features, which include hypotheses about crustal loading mechanisms due to deeper mantle processes, remain an active area of seismologic research. But experience has shown that only those tectonic features/hypotheses having a significant probability of being seismogenic (Pa greater than about 0.5) will have hazard significance. Therefore, the evaluation of tectonic features/hypotheses with low Pa can only be represented in the regional characterization of seismotectonic source zones. Any consideration of local tectonic features would be part of a site-specific refinement to the regional SSC model.
- Geologic observations of paleoearthquakes are now largely accepted within the technical community and can be viewed as an extension of the observed seismicity record back in time. Further, these earthquakes have been shown to have a profound effect on hazard estimates for many sites within the CEUS. Therefore, they must be included explicitly in the seismic source model for the CEUS. However, the uncertainties in the location, magnitude, and recurrence of these earthquakes are evaluated differently from those of the historical and instrumental seismicity record. As a result, the CEUS-SSC model should provide for paleoseismic earthquakes explicitly, but should also provide for addressing their uncertainties in a manner different from the rest of the observed seismicity catalog.
- The logic structure for the SSC model, represented by a master logic tree, should provide alternative approaches and conceptual models for our current understanding of the constraints on the location, size, and recurrence of future earthquakes. For clarity and efficiency, the logic tree should start with the most basic descriptions of seismic sources and should gain complexity only as needed to represent specific hypotheses and data sets that have hazard significance. In this way, unnecessarily complex source models will be avoided, such as those that depict a large number of tectonic features, none of which have a significant probability of being seismogenic.
4.1.3.3 Criteria for Defining Seismic Sources
Embarking on the development of a new SSC for the CEUS demanded that attention be given to the experience gained from similar efforts over the past few decades, in terms of both the development of new data and tools and the experience with issues of most significance to hazard at annual frequencies of interest for nuclear power plants. On the one hand, geologic and geophysical studies of the crust since the 1980s have provided little new information about tectonic features and the geologic history of the region that may have a bearing on evaluation of seismic hazards; a possible exception, however, is the improved understanding of the Illinois Basin Extended Basement and its features. On the other hand, paleoliquefaction studies have been useful in defining and characterizing seismic source zones.
The methodology needed to be consistent with the seismotectonic setting of the CEUS and our current knowledge base for assessing the locations, sizes, and rates of future earthquakes. For example, we currently lack a clear definition of the causative faults giving rise to the observed seismicity, so applying a methodology that relies on knowledge of fault location and behavior would not be appropriate. Similarly, geodynamic data on contemporary crustal strain are currently limited in their duration and spatial extent; in addition, available physical models are unable to make a unique association between geodetic strain and earthquake processes (NEPEC, 2011). Therefore, although such data may be useful in assisting with the evaluation of seismic source characteristics, the methodology should not rely on knowledge of the relationship between short-term crustal strain data and future earthquake characteristics.
Various authors over time have defined seismic sources for purposes of PSHA in different ways. For example:
- “Sources are explicitly defined as being of uniform earthquake potential; that is, the chance of an earthquake of a given size is the same throughout the source.” (Reiter, 1990)
- “[A seismic source is] a region of the earth’s crust that is assumed for PSHA to have
relatively uniform seismic source characteristics.” (Budnitz et al., 1997)
- “A seismic source is a volume of the earth’s crust that has the same earthquake potential as defined by the size of events that may be generated.” (BC Hydro, 2008)
A common theme in these definitions is a degree of “uniform” earthquake potential or characteristics, although exactly what this means is not clear or varies with the application. Early in the history of PSHA, the Mmax (and associated uncertainty) and recurrence rates (expressed as a- and b-values) within identified seismic sources were assumed to be “uniform.” “Uniform” in this case meant the same throughout the source without spatial variation. Since then, a number of approaches have been developed to express the spatial variation of recurrence parameters. For example, the EPRI-SOG project provided for spatial variation of a- and b-values at the scale of one-degree cells (~100 km [~62 mi.] dimensions), and the USGS national hazard maps utilize a Gaussian smoothing kernel to express spatial variations in a-values. Thus far, the spatial variation in Mmax has only been expressed by the identification of separate sources (including fault sources within areal source zones), and a strong technical basis for spatial variations of Mmax within source zones has not been established.
Given the evolution of approaches to identifying seismic sources, it is appropriate to provide a set of criteria and the logic for their application in the CEUS-SSC Project. In the project, unique seismic sources are defined to account for distinct differences in the following criteria:
- Earthquake recurrence rate
- Maximum earthquake magnitude (Mmax)
- Expected future earthquake characteristics (e.g., style of faulting, rupture orientation, depth
distribution)
- Probability of activity of tectonic feature(s)
Rather than treat these criteria as operating simultaneously or without priority, the CEUS-SSC methodology works through them sequentially. Their sequence represents their relative significance to seismic hazard results, with earthquake recurrence rate being most important and the probability of activity having lesser impact on calculated hazard results. Further, because each criterion adds complexity to the seismic source model, it is applied only if its application would lead to hazard-significant changes in the model. In this way, the model becomes only as complex as required by the available data and information.
Examples will assist in illustrating the notion of progressively applying the seismic source criteria. To begin, consider the entire CEUS study region and the first criterion of differences in earthquake recurrence rate. In general, the record of past earthquakes is obtained from the historical/instrumental catalog and from the paleoseismic record of prehistoric earthquakes. For the CEUS-SSC Project, RLME sources are the locations of repeated (more than one) large-magnitude (M ≥ 6.5) earthquakes, and paleoseismic evidence is used to define the source’s recurrence rate (see Section 4.4.1.1 for further discussion of RLME sources). This is an example of identifying distinct seismic sources based on differences in recurrence.
Spatial smoothing of the recurrence rate (a- and b-values) based on observed seismicity accounts for the spatial variation in rate. The approach used in the CEUS-SSC is a refinement of that used in the EPRI-SOG project. Conceptually, the smoothed seismicity model is the least complex seismic source representation. Embedded within the concept of spatial smoothing is the notion of spatial stationarity; that is, the pattern of past earthquakes is a predictor of the pattern of future earthquakes. Studies of seismicity in the CEUS have concluded that this is a reasonable interpretation (Kafka, 2007, 2009). Further, because the historical record of observed earthquakes is relatively short (about 200 years in most of the CEUS) relative to the recurrence intervals for large-magnitude earthquakes, there is an assumption that the spatial distribution of observed smaller-magnitude earthquakes constrains the spatial distribution of larger-magnitude earthquakes. The use of spatial smoothing to represent earthquake recurrence, together with RLME sources, means that there may not be a need to identify seismic source boundaries within a region due to recurrence differences.
After spatial variations in rate have been established using smoothing, then the CEUS can be subdivided to account for differences in Mmax. Current approaches to assessing Mmax within SCRs such as the CEUS are based on analogies to domains having similar tectonic characteristics. The EPRI Mmax project (Johnston et al., 1994) presented a Bayesian approach to assessing Mmax that establishes prior distributions of Mmax for two domains: extended crust (defined as having undergone major extension in Paleozoic and younger time) and non-extended crust. These prior distributions are modified by a likelihood function that reflects the earthquake counts within a seismic source and is truncated at the low-magnitude end by the largest observed earthquake within the source of interest. The SCR database and analysis of the data given in Johnston et al. (1994) were updated as part of the CEUS-SSC Project (see the discussion in Section 5.2.1.1).
The results of the data reanalysis suggest that there is only a weak statistical basis for separation of the SCR data to establish a prior distribution on Mmax. As a result, the CEUS-SSC model invokes either a single prior distribution that is applicable to the entire CEUS-SSC study region, or two prior distributions: one that is based on Mesozoic-and-younger extension and one that is based on non-extended regions or older extended regions. In the latter case, a seismic source boundary is drawn (including uncertainty) to separate the regions of Mesozoic-and-younger extension from the remainder of the study area. This is an example of a seismic source being defined on the basis of Mmax differences.
From variations in recurrence and Mmax, the next criterion for subdividing the CEUS is expected significant differences in future earthquake characteristics, such as their depth distribution, style of faulting, and expected orientation of earthquake ruptures (strike and dip). In seismic hazard models, future earthquakes are modeled as having finite dimension, magnitude-dependent rupture dimensions, orientations, and depth extent. This is because these characteristics are important to modern ground-motion prediction equations, including those that will be developed for the CEUS region as part of the ongoing Next Generation Attenuation East (NGA-East) project (PEER, 2010). To accommodate these assessments, the CEUS study region was subdivided into seismotectonic zones having comparable characteristics. These subdivisions may also have implications for Mmax assessments in that the likelihood function varies with the size of the largest observed earthquake for the source of interest. Within these subdivisions, spatial smoothing of seismicity is carried out to express the variation of recurrence rate spatially.
A final assessment that can be considered is the identification of particular tectonic features that have significant potential to localize seismicity; that is, they are assessed to have a Pa that is greater than about 0.5. These might be associated with a paleoearthquake, smaller-magnitude earthquakes, or they might have geologic indicators of activity. In the cases where potentially seismogenic tectonic features are identified, it is necessary to consider the relationship between the feature and the local background within which the feature lies. For example, if the feature has a Pa less than 1.0, then there is a finite probability (1–Pa) that the feature is not seismogenic and does not localize seismicity. In that case, the background zone would need to be identified. Likewise, in the case where the feature is judged to be seismogenic, the earthquakes that should be assumed to be associated with the feature need to be identified so that recurrence rate for the feature and the background zone can be calculated. The CEUS-SSC Project identified very few local tectonic features with clear and compelling reported evidence of activity and these are the RLME fault sources (e.g., the Meers fault and Cheraw fault). However, because the CEUS-SSC model is a regional model, any site-specific use of the model will need to consider whether any local evidence for tectonic feature activity might exist and, if so, refine the model locally.
The basis for the assessment of the recency of fault displacement and the potential for Quaternary activity is the comprehensive study conducted by Crone and Wheeler (2000), who place each feature into Classes A through D depending on what is known about the feature’s geologic evidence for Quaternary activity. The inclusion of faults that only have a high probability of activity in the CEUS-SSC model does not preclude, however, the need to consider local site-specific data and evidence for the potential activity of tectonic features on a local scale. It is anticipated that the required site-specific data collection studies for a nuclear facility will provide the basis for identifying potential local seismic sources and, if necessary, local refinements to the CEUS-SSC model.
The application of the criteria for identifying seismic sources results in the suite of seismic sources given in the CEUS-SSC model. A summary of the criteria that resulted in the identification of each of the seismic sources is given in Table 4.1.3-1. A detailed description of the application of the criteria to each source is given in the “Basis for Defining Seismotectonic Zone” sections in Chapter 7 (e.g., Sections 7.3.6.2 and 7.3.7.2). In addition, the bases for defining the RLME sources and the Mmax zones are given in applicable sections of Chapter 6 (e.g., Section 6.2.1). In those cases where alternative source geometries are included in the SSC model, a discussion of the alternatives and the basis for the weights assigned to each alternative are also given in the applicable sections of Chapters 6 and 7.
To represent the uncertainties in the seismic source identification process, both a master logic tree and individual seismic source logic trees were constructed. These are discussed below.
The master logic tree establishes the framework for the entire seismic source model. It identifies the alternative approaches and conceptual models that will be used and establishes the relative weights assigned to the main alternatives. By laying out the alternatives at the start, the subsequent detailed source evaluations will each be conducted within a framework that ensures consistency across all sources. Likewise, the sum total of the source evaluations will be logically combined in such a way as to avoid double-counting and provide for meaningful weighted combinations. In this section, the discussion of the master logic tree is followed by a description of the major elements of the logic trees that describe the various seismic sources. The detailed discussions of the individual seismic sources and the characterizations in their logic trees are given in Sections 6 and 7 of this report.
4.2.1 Description of Logic Tree Elements
Using the criteria given in Section 4.1.3.3 and the associated conceptual basis, a master logic tree was developed that provides a framework for all of the seismic source evaluations in the CEUS-SSC (Figure 4.2.1-1). The basic structure of the logic tree has been developed to include the simplest representation of seismic sources (smoothing of observed seismicity with subdivisions of the CEUS related to recurrence and Mmax) as well as more complex subdivisions to account for differences in the characteristics of future earthquakes. Accordingly, the first-order branches of the tree address the basic conceptual models related to the approaches; these are followed by branches that represent the uncertainties in the implementation of each approach.
The first assessment on the master logic tree (Figure 4.2.1-1), represented by the first node, is the choice between two conceptual models used to assess the spatial and temporal distribution of future seismicity. The application of the seismic source criteria given in Section 4.1.3.3 leads to the identification of RLME sources based on differences in earthquake recurrence (from paleoseismic evidence) from the “background” zones within which they lie. RLME sources are identified based on well-defined evidence for Late Quaternary or Holocene RLMEs. Thus the RLME sources are present for all seismic source interpretations.
The “Mmax zones” model involves identifying alternative configurations based on differences in the prior distribution of Mmax using the Bayesian Mmax approach (see Section 5.2.1.1). Accordingly, the CEUS-SSC study region is either subdivided according to evidence of Mesozoic and younger extension (with associated uncertainties in the location of the boundary) or not subdivided. In this model, the spatial variation of recurrence parameters is based on spatial smoothing of observed earthquakes. The “seismotectonic zones” model also includes the concept of subdividing the region according to differences in the prior Mmax distributions, and identifies seismic sources based on spatial variations in the characteristics of future earthquakes (the third criterion identified in Section 4.1.3.3).
In addition to the RLME sources, the region is divided into seismotectonic zones that provide for differences in expected future earthquake characteristics. For example, differences in the style of faulting, strike of ruptures, and depth distribution of future earthquakes can be accommodated in the “seismotectonic zones” model. The model also accommodates any differences in Mmax among the seismotectonic zones due to differences in the size of the largest observed earthquakes; Sections 5.2.1.1 and 5.2.1.2 describe the influence of the largest observed earthquakes on the Mmax estimates.
The weights assigned to the “Mmax zones” and “seismotectonic zones” branches reflect the relative preference for the alternative approaches for characterizing the future spatial and temporal distribution of earthquakes and their characteristics, given the available data for the CEUS. The two models are quite similar in many respects. They both include RLME sources as independent sources defined by paleoseismic evidence for the size and recurrence rate for the RLME earthquakes. Moreover, both allow spatial variation of recurrence parameters by smoothing within seismic source zones (see Section 5.3.2 for a discussion of spatial smoothing). The key difference between the two models is in their ability to include and represent information related to the characteristics of future earthquakes. The “Mmax zones” model is based on average or “default” characteristics that are representative of the the entire study region (Table 5.4-1), whereas the “seismotectonic zones” model can include information that allows for an assessment of spatial variations of future earthquake characteristics at a scale that is appropriate to a regional SSC model (see Table 5.4-2 for the characteristics of each seismotectonic zone). A higher weight (0.6) is assigned to the seismotectonic zones branch than to the Mmax zones branch (0.4) because the seismotectonic zones approach allows for more relevant information on the characteristics of future earthquakes to be included in the model. While many of the characteristics of the seismotectonic zones are uncertain, such as the locations of the source boundaries and the characteristics of future earthquake ruptures, they are still judged to provide a better description of the applicable source characteristics.
Early in the project, as part of the SSC sensitivity model, a third conceptual model was considered that would be even simpler conceptually than the Mmax zones model. This model was called the “zoneless” model and it postulated that all earthquakes-both those defined from the historical record and those defined from paleoseismic evidence-would be subject to spatial smoothing. As such, the model would not need to invoke any source zone boundaries, including those that identify RLME sources. With further consideration, however, it was found that the model cannot be applied with confidence given our present knowledge. This is because the spatial smoothing approach is actually smoothing the recurrence parameters a and b, which require that the record be complete over a given time interval. Completeness adjustments can readily be made for the historical record, but there is not sufficient information in the paleoseismic record to make the same type of completeness adjustments. The current spatial distribution of paleoseismic investigations is decidedly non-uniform. Some areas have been investigated in detail, and estimates of the completeness of the record locally are possible, but other areas have not been subject to searches for paleoseismic evidence at all. Until systematic searches for paleoseismic evidence are conducted such that the completeness of the record can be assessed and corrected for, it is not possible to exercise the “zoneless” model, and it has been dropped from the CEUS-SSC model. It is mentioned here, however, in anticipation that future work will allow its incorporation into SSC models.
Given either the Mmax zones or seismotectonic zones branches of the master logic tree, certain source characteristics are defined in the subsequent parts of the logic tree. A detailed discussion is given in Section 5 of the various approaches used in the CEUS-SSC Project to characterize the Mmax (Section 5.2), earthquake recurrence (Section 5.3), and future earthquake characteristics (Section 5.4). Here we present the major elements of the master logic tree and discuss why they are included. The discussion in this section also includes the relative weights assigned to assessments that are not source-specific. The source-specific assessments for RLME sources are given in Section 6.1, for Mmax zones in Section 6.2, and for seismotectonic zones in Section 7.3.
RLME sources are identified and characterized in either the Mmax zones or the seismotectonic zones branches. The logic tree that describes the RLME source characteristics is given on Figure 4.2.2-1, which shows an example tree for the Marianna RLME source. Figure 4.2.2-2 identifies the RLME sources, which are listed in Table 4.2.2-1. In this section of the report, the characteristics are described generically without reference to any particular RLME source. Individual RLME source characteristics are described in Section 6.1.
The first node of the logic tree for RLME sources (Figure 4.2.2-1) deals with the issue of temporal clustering of large-magnitude earthquakes. Many seismic sources, especially those within SCRs, display evidence of clustering through time such that the recurrence rates may be elevated for several seismic cycles during a cluster, followed by much longer time intervals. This behavior can be modeled by identifying two rates: the within-cluster rate and the out-of-cluster rate. The SSC model resulting from the CEUS-SSC Project will be useful for engineering applications that will entail up to approximately the next 50 years;1 for this reason, it is important to assess whether the source is currently (i.e., over approximately the next 50 years) within or out of a cluster such that the within-cluster or out-of-cluster rate is applicable. This is the first assessment in the RLME source logic tree.
The second node of the logic tree is the assessment of the nature of the localizing tectonic feature for the RLMEs. In some cases the source will be modeled as a fault source; in other cases the existing data will not allow for a clear definition of causative faults, and some type of areal source zone will be used. Alternative geometries are then defined at the third node of the tree for the localizing tectonic feature(s).
The fourth and fifth nodes of the tree provide information regarding the rupture characteristics for future earthquakes within the RLME source. As discussed in Section 5.4 and shown in Table 5.4-1, a “default” set of characteristics were developed for the entire study region, and the assessments made by the TI Team for individual seismic sources could either adopt the default characteristics or, if sufficient data were available to do so, specify source-specific characteristics. Source-specific characteristics are included on the logic tree and are shown in Table 5.4-2. Shown are seismogenic crustal thickness, rupture orientation, and source boundary characteristics. Seismogenic crustal thickness can be important in the assessment of distance from ruptures for ground-motion prediction equations, as well as in calculations of seismic moment rate from geologic slip rates. Also, the dimensions of rupture are magnitude dependent (Section 5.4), and finite ruptures, using the assessed rupture orientations and downdip dimensions, are modeled for purposes of the hazard calculations. The hazard model assumes that the epicenters of all earthquakes will occur within the seismic source, although the seismic source boundary characteristics are assessed for whether the rupture can cross the source boundary (termed a “leaky” boundary) or must remain within the boundary (“strict” boundary). Not shown on the tree is the assessment of the style of faulting for the source.
1 Note that 50 years is the approximate lifetime of nuclear facilities and is used in this context as the time period of interest for assessing within-cluster or out-of-cluster rates. There is no implication that the lifetime of the CEUS-SSC model is 50 years.
The sixth node of the logic tree expresses the estimates of the RLME magnitudes. Because most of the evidence for the RLMEs in the CEUS comes from paleoseismic data, there can be significant uncertainty in the size of the earthquakes. There are two components to this assessment: an aleatory component that expresses the variations in the size of the RLME eventto-event, and an epistemic component that expresses the uncertainty in the average size of the RLME. The epistemic component is given in the logic tree, and the aleatory component is assumed to be plus or minus 0.25 magnitude units about the mean unless there is source-specific information that suggests otherwise. The value of 0.25 magnitude is judged to be appropriate based on observations of the repeated sizes of paleoearthquakes in well-studied areas.
The seventh node of the logic tree is the recurrence method and differs depending on whether the “in-cluster” or “out-of-cluster” branch is being followed. Given the “in-cluster” branch, approaches to estimating recurrence include either interevent times (recurrence intervals) or slip rates. In either case, the data should be those that are applicable to the present cluster and that would apply for the future period of interest of about 50 years. Given the “out-of-cluster” branch, the assessments of recurrence should again focus on the applicable recurrence information that would apply to the future period of interest.
The eighth “events/data” node of the logic tree expresses the data that are used in the recurrence assessment for the RLME source. In most cases, this is an assessment of the dates of past earthquakes, which includes the uncertainty in the timing of earthquakes given the available paleoseismic data. In other cases, an assessment is made of the number of events that have occurred over a particular time interval. The approaches taken in the estimation of RLME recurrence are described in Section 5.3.3.
The earthquake occurrence model in the ninth node of the logic tree expresses the approach that is used to model the temporal occurrence of earthquakes. Two alternative models are considered, depending on the availability of data for the RLME source of interest. A Poisson model assumes that earthquakes occur in a temporally random way that is defined simply by a mean recurrence rate without regard to the time elapsed since the last earthquake. The Poisson model is the most commonly used in PSHA because of the minimal number of parameters that must be constrained. An alternative model is the renewal model (strictly, a Brownian passage time, or BPT, model is used [see Section 5.3.3.2]), which requires information not only on the mean recurrence rate, but also on the aperiodicity factor (alpha) and the time elapsed since the most recent earthquake. The model is based on a strain accumulation and release physical model that is most applicable to a fault source. To be compatible with common PSHA models, the resulting recurrence rates for both the Poisson and renewal models are expressed as equivalent annual frequencies, as shown in the last node of the RLME source logic tree.
As implied by the name, the Mmax zones model considers possible subdivisions of the CEUS based on considerations of Mmax. As discussed in Section 5.2, two approaches to estimating Mmax are used in the CEUS-SSC Project:
- The Bayesian approach (Johnston et al., 1994), in which prior distributions are based on statistical analyses of tectonically analogous domains to the CEUS worldwide, and likelihood functions are derived from the number and size of earthquakes that occur within the seismic source of interest.
- The Kijko (2004) approach, in which the statistics of observed earthquakes within the source of interest are used to estimate Mmax.
The two approaches are similar in the use of observed seismicity data within the source of interest, but they differ in the use of a prior distribution in the Bayesian approach. For the CEUS-SSC Project, the global database of tectonically analogous earthquakes was updated from the Johnston et al. (1994) study and the prior distributions from that study were reassessed. As discussed in Section 5.2, the statistics do not strongly define the prior distributions. This means that there are no unique tectonic characteristics that strongly correlate with maximum earthquake size. Past studies using the database have suggested that a difference exists between sources having Paleozoic and younger extension and those that do not. However, the analysis conducted for this project does not support that view, but suggests that the only potentially significant difference is between sources having Mesozoic and younger extension and those that do not.
Based on the analyses conducted for Mmax, two alternative models define the first branch of the Mmax zones logic tree (Figure 4.2.3-1): a branch that represents the entire CEUS-SSC study region by a single prior distribution, and a branch that calls for the separation of Mesozoic and younger extended regions from those that do not display such evidence. The available evidence and statistical analyses of the global SCR database (Section 5.2) carried out as part of the CEUS-SSC Project suggests that the separation into Mesozoic and younger sources is significant, but only marginally so. Therefore, the approach that uses the separation is preferred (0.6) over the approach that does not recognize a separation (0.4), although the preference is not large, given the marginal statistical significance.
The second node of the Mmax zones logic tree, which applies only to the Mesozoic and younger separation branch, considers the uncertainty in the location of the boundary between Mesozoic and younger regions and those that do not show evidence of such extension. As discussed in Section 6.2, there is stronger technical support for the “narrow” interpretation in the available data than the “wide” interpretation.Two alternative locations are considered, with higher weight (0.8) given to the narrow interpretation than the wide interpretation (0.2). These two alternative locations of the boundary are shown on Figures 4.2.3-2 and 4.2.3-3.
The third node addresses the issue of the weight assigned to various magnitudes in the estimation of seismicity parameters for the seismic source zones. The three alternatives, Cases A, B, and E are discussed in Section 5.3.2.2.1, along with the bases for the weights assigned to the alternatives.
The remaining assessments of the logic tree are a function of the region that is being characterized, which is either the entire study region, the Mesozoic extended-wide, the Mesozoic extended-narrow, or the non-Mesozoic extended regions.
Similar to the RLME sources, the next assessments in the logic tree are related to the characteristics of future earthquake ruptures. The first assessment is seismogenic crustal thickness, which controls the downdip extent of ruptures, and the second is rupture orientation and boundary characteristics. Given the large extent of the regions of interest in the Mmax zones model, the characteristics of future ruptures are those given in the “default” set of characteristics for the entire study region (see Section 5.4).
The next level of the logic tree addresses the approach used for assessing seismicity rates and their spatial distribution. Allowing both the a-value and the b-value to vary spatially is the selected approach. Seismicity parameters are estimated for ¼° × ¼° cells using an update of the approach developed in EPRI-SOG.
The “degree of smoothing” level of the logic tree addresses the degree of smoothing applied in the seismicity parameter estimation in each source region. An “objective” approach is used to select the degree of smoothing, as discussed in Section 5.3.2.4.
The next level of the logic tree addresses the seismicity parameter epistemic uncertainty. The seismicity parameter distributions for the “variable a and b” approach are represented by eight alternative spatial distributions developed by simulation from the fitted parameter distributions (Section 5.3.2.5).
The final level of the logic tree addresses the uncertainty in the maximum magnitude for each region. This assessment includes uncertainty in the basic approach to estimating Mmax as well as uncertainties with a given approach. The two alternative approaches estimating Mmax are the Bayesian approach developed in Johnston et al. (1994) with updated prior distributions developed in this project, and the Kijko (2004) approach that uses the numbers and magnitudes of observed earthquakes directly without a prior distribution. As discussed in Section 5.2.1.3, the relative weights applied to the two approaches are source-specific and region-specific and are related directly to the p-value derived from the Kijko approach. Given the Bayesian approach, two prior distributions are considered, depending on the assessment in the first node of the logic tree. If the region is not subdivided (the “no” branch on the first node), then a single composite prior distribution is used. If a separation is made between Mesozoic and younger extension and non-Mesozoic extension, then the appropriate prior distributions for those regions are used for the Mmax estimates.
4.2.4 Seismotectonic Zones Branch
The seismotectonic zones identified for the CEUS-SSC model are listed in Table 4.2.4-1. The logic tree for the seismotectonic zones branch of the master logic tree is shown on Figure 4.2.4-1, and the maps of the seismotectonic zones are shown on Figures 4.2.4-2 through 4.2.4-5. Following the “seismotectonic zones” branch of the master logic tree, the first assessment is the uncertainty in the western boundary of the Paleozoic Extended Crust seismotectonic zone. The two alternatives are the narrow interpretation (0.8) and the wide interpretation (0.2). As discussed in Section 7.3.4, there is significantly more technical support for the location of the boundary in the narrow case.
The second node of the logic tree addresses the uncertainty in the eastern extent of the Reelfoot Rift zone-whether or not it includes the Rough Creek Graben. These two logic tree branches lead to the four alternative seismotectonic zonation configurations shown on Figures 4.2.4-2 through 4.2.4-5. The discussion of this assessment and the associated weights is given in Section
7.3.6.3.
The third node of the logic tree represents the uncertainty in the issue of the weight assigned to various magnitudes in estimating seismicity parameters for the seismotectonic zones. The assessment is the same as that given in the Mmax zones branch and is discussed in Section
5.3.2.2.1
The next element of the tree (which is not a node but a listing) identifies the various seismotectonic zones included in the CEUS-SSC model, which are given in Table 4.1.3-1.
Similar to the RLME sources, the next assessments in the logic tree are related to the characteristics of future earthquake ruptures. The first assessment is seismogenic crustal thickness, which controls the downdip extent of ruptures, and the second is rupture orientation and boundary characteristics. The characteristics of future ruptures are discussed in the “default” set of characteristics for the entire study region (see Section 5.4); each seismotectonic zone is assigned a set of characteristics based on the applicable data specific to that zone.
The next level of the logic tree addresses the approach used for assessing seismicity rates and their spatial distribution. Allowing both the a-value and the b-value to vary spatially is the selected approach. Seismicity parameters are estimated for ¼° × ¼° cells using an update of the approach developed in EPRI-SOG.
The “degree of smoothing” level of the logic tree addresses the degree of smoothing applied in the seismicity parameter estimation in each seismotectonic zone. An “objective” approach is used to select the degree of smoothing, as discussed in Section 5.3.2.4.
The next level of the logic tree addresses the seismicity parameter epistemic uncertainty. The seismicity parameter distributions for the “variable a and b” approach are represented by eight alternative spatial distributions developed by simulation from the fitted parameter distributions (Section 5.3.2.5).
The final level of the logic tree addresses the uncertainty in the maximum magnitude for each seismotectonic zone. This assessment includes uncertainty in the basic approach to estimating Mmax as well as uncertainties for a given approach. The two alternative approaches estimating Mmax are the Bayesian approach developed by Johnston et al. (1994) with updated prior distributions developed in this project, and the Kijko (2004) approach that uses the numbers and magnitudes of observed earthquakes directly without a prior distribution. As discussed in Section 5.2, the relative weights applied to the two approaches are source-specific and region-specific and are related directly to the p-value derived from the Kijko approach. Given the Bayesian approach, two options are available regarding prior distributions: a “composite” prior that is based on the entire SCR data set, or two priors that are based on Mesozoic or younger extension and non-Mesozoic or younger extension. As discussed in Section 5.2, the relative weight assigned to the composite distribution is 0.4 and the relative weight of the two-prior option is 0.6. These relative weights are assigned to each seismotectonic source, but given the two-prior option, a source-specific assessment must be made as to whether the zone lies within a Mesozoic or younger extended region.
Table 4.1.2-1
Sample table indicating particular types of data that can be considered in the identification and characterization of seismic sources (Table 2, ANSI/ANS-2.27-2008)
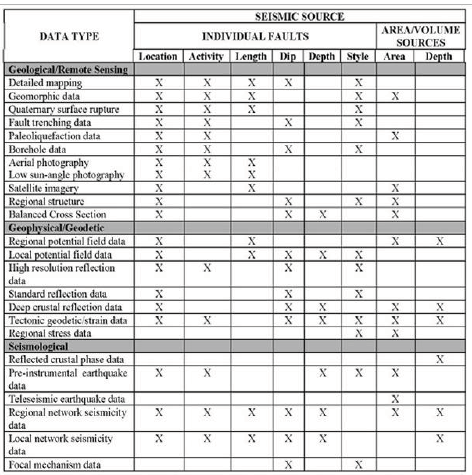
Table 4.1.2-2
Sample table identifying the types of data that can be considered for characterizing different types of seismic sources, and an evaluation of the relative usefulness or credibility of the various data types (Budnitz et al., 1997)
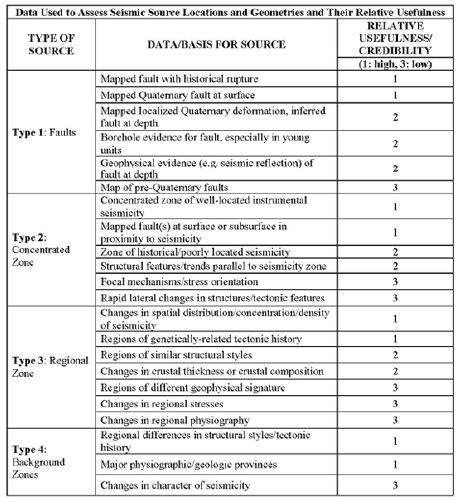
Table 4.1.2-3
Table showing the “generic” (not source-specific) evaluation of data to address indicators of a unique seismic source. The table
indicates the TI Team’s assessment of the types of data that can be used to address the indicators and their relative usefulness
| Indicators of a Potential Seismic Source | Usefulness of Indicator in Defining Seismic Sources 5= High 1= Low |
Data to Address Indicator | Relative Usefulness of Data in Addressing Indicator 5= High 1= Low |
Notes: Source Aspect (Temporal, Spatial) |
|---|---|---|---|---|
| Paleoseismic indicators of M > 5 earthquakes | 5 |
Paleoliquefaction evidence | 4 |
Temporal, spatial |
| Quaternary faulting | 5 |
|||
| Quaternary deformation | 3 |
|||
| High strain rates in contemporary tectonic setting | 4 |
Tectonic geodetic strain data | 4 |
Temporal, perhaps spatial |
| Geologic indicators of recent strain (e.g., Quaternary) | 5 |
|||
| Variations in stress/strain orientations | 3 |
Tectonic geodetic strain data | 3 |
Spatial |
| Zones of weakness, including both crustal and mantle (including hotspot tracks and lithospheric upwelling) | 1 |
Tectonic geodetic strain data/modeling | 1 |
Spatial |
| Geophysical evidence of mantle anomalies (e.g., tomography, heat flow, concentration of heat-producing elements) | 3 |
|||
| Consideration of rheology based on rock types/petrology | 2 |
|||
| Geologic mapping | 3 |
|||
| Evidence for recent and/or repeated reactivation of preexisting structures (Note: General types of structures should be identified with a focus on those that may contain faults of sufficient dimension to cause M > 5 earthquakes.) | (see below) |
(see below) | (see below) |
(see below) |
| (1) Cratons | 1 |
Geologic mapping | 2 |
Spatial |
| Potential field geophysics (magnetic, gravity) | 2 |
|||
| Historical and instrumental seismicity | 2 |
|||
| Deep crustal seismic profiles | 3 |
|||
| Compilations of historical analogues | 2 |
|||
| (2) Extended Margins-and age (Mesozoic and younger) | 1 |
Geologic mapping | 2 |
Spatial |
| Potential field geophysics (magnetic, gravity) | 2 |
|||
| Historical and instrumental seismicity | 2 |
|||
| Deep crustal seismic profiles | 3 |
|||
| Compilations of historical analogues | 2 |
|||
| (3) Rifted Margins-and age (Mesozoic and younger) | 2 |
Geologic mapping | 2 |
Spatial |
| Potential field geophysics (magnetic, gravity) | 2 |
|||
| Historical and instrumental seismicity | 2 |
|||
| Deep crustal seismic profiles | 3 |
|||
| Compilations of historical analogues | 2 |
|||
| (4) Rift Basins | 2
|
Geologic mapping | 2 |
Spatial |
| Potential field geophysics (magnetic, gravity) | 2 |
|||
| Historical and instrumental seismicity | 2 |
|||
| Deep crustal seismic profiles | 3 |
|||
| Compilations of historical analogues | 2 |
|||
| (5) Failed Rift (Paleozoic and younger) | 1 |
Geologic mapping | 2 |
Spatial |
| Potential field geophysics (magnetic, gravity) | 2 |
|||
| Historical and instrumental seismicity | 2 |
|||
| Deep crustal seismic profiles | 3 |
|||
| Compilations of historical analogues | 2 |
|||
| Cold strong crust | 1 |
Heat flow | 2 |
Spatial |
| Geophysical modeling of mantle processes (e.g., tomography) | 3 |
|||
| Geologic evidence for potential zones of stress concentration/amplification | 2–3 |
Analysis of instrumental seismicity data (depths, focal mechanisms) | 2 |
Spatial |
| Consideration of rheological contrasts based on geologic mapping and modeling (mafic plutons, intersecting faults) | 2 |
|||
| Orientation of structures relative to underlying stress field (either favorable or unfavorable) | 2 |
Analysis of instrumental seismicity data (depths, focal mechanisms) | 2 |
Spatial |
| Geologic mapping and geophysical interpretations of structures at depth | 2 |
|||
| Local loading mechanisms (as stress concentrators) | 1 |
Geologic mapping | 2 |
Spatial |
| Detailed topographic analysis | 2 |
|||
| Isostatic analyses (sediment load/denudation, glacial forebulge, or rebound) | 3 |
|||
| Evidence of geologically recent fault displacement | 5 |
Mapped fault with historical rupture | 5 |
Spatial, temporal |
| Mapped Quaternary fault at surface | 5 |
|||
| Mapped localized Quaternary deformation, inferred fault at depth | 4 |
|||
| High-resolution seismic reflection or borehole evidence for fault, especially in young units | 3 |
|||
| Fault having significant dimensions | 1 |
Geophysical evidence (e.g., seismic reflection) of fault at depth | 3 |
Spatial |
| Map of pre-Quaternary faults | 3 |
|||
| Concentrated zone of observed seismicity | 4 |
Well-located instrumental seismicity | 5 |
Spatial, Temporal |
| Fault(s) mapped at surface or subsurface in proximity to seismicity; alignments parallel to structure | 3 |
|||
| Historical seismicity | 3 |
|||
| Focal mechanisms/stress orientation | 2 |
|||
| Rapid lateral changes in structures/tectonic features/observed seismicity | 3–4 |
Historical and instrumental seismicity showing changes in spatial distribution/concentration/density of seismicity | 3 |
Spatial |
| Geologic/tectonic maps showing regions of genetically related tectonic history; similar structural styles | 3 |
|||
| Geophysical maps showing changes in crustal thickness or crustal composition | 3 |
|||
| Regions of different geophysical signature | 3 |
|||
| Stress indicators showing changes in regional stresses (e.g., compressional to tensional; orientation of horizontal stress directions) | 2 |
|||
| Changes in regional physiography (e.g., fall line) | 2 |
|||
| Regional or local strain energy buildup following larger (M > 7) earthquakes (e.g., New Madrid earthquakes trigger earthquakes to the north) | Note: The occurrence of the M > 7 earthquake would define the unique seismic source; current temporal methods do not account for triggering of adjacent earthquakes. | Temporal |
||
| Stress shadows following large earthquakes | Note: Occurrence of large earthquakes would be considered in defining seismic source; real-time model would be needed to account for time-dependent temporal behavior; this indicator has more applicability for modeling of stress interactions among faults in WUS. | Spatial, temporal |
||
| Regional variations in expected Mmax or recurrence (background zones) | Note: These are applicable criteria, but they are based on a derivative product (Mmax or recurrence) and not data per se. | Spatial, temporal |
1 Each indicator is assumed to be known with certainty.
2 It is assumed that high-quality data exist of the type identified.
3 Could be accounted for using spatial smoothing, thus not requiring a source boundary.
Table 4.1.2-4
Example of Data Evaluation Table for the Illinois Basin–Extended Basement Zone (IBEB)
| Data/References | Quality (1=low, 5=high) |
Notes on Quality of Data | Source Considered | Used in SSC and Reliance Level (0=no, 5=high) |
Discussion of Data Use | In GIS Database |
|---|---|---|---|---|---|---|
| Instrumental Seismicity | ||||||
| CEUS-SSC earthquake catalog | 5 |
Comprehensive catalog; includes magnitude conversions and uncertainty assessments. | IBEB |
5 |
Used to evaluate recurrence parameters. | Y |
| Hamburger et al. (2008) | 3 |
Abstract | IBEB |
4 |
Style of faulting and future earthquake characteristics-Reactivation of structures in contemporary stress regime in Illinois basin region-04:30 CDT, April 18, 2008, M 5.4 earthquake, located near New Harmony fault at depth of ~14 km (~9 mi.). | Y |
| Withers et al. (2009) | 3 |
Abstract-citing preliminary analysis. | IBEB |
4 |
Style of faulting and future earthquake characteristics-Reactivation of structures in contemporary stress regime in Illinois basin region-April 18, 2008, M w 5.2 (M w 5.4 GCMT [http://www.global cmt.org]) Mt. Carmel, Illinois, earthquake. Largest event in 20 years in Wabash Valley seismic zone. | Y |
Table 4.1.2-5
Example of Data Summary Table for the Extended Continental Crust–Atlantic Margin (ECC-AM) and Atlantic Highly Extended Crust (AHEX) Zones
| Citation | Title | Description and Relevance to SSC |
|---|---|---|
| General for Region | ||
|
Austin et al. (1990) |
Crustal Structure of the Southeast Georgia Embayment-Carolina Trough: Preliminary Results of a Composite Seismic Image of a Continental Suture(?) and a Volcanic Passive Margin | The authors use multichannel seismic-reflection data to image the Carolina platform and conclude that observed magnetic anomaly in this region is the product of Mesozoic rifting processes, not Paleozoic collision. |
Bird et al. (2005) |
Gulf of Mexico Tectonic History: Hotspot Tracks, Crustal Boundaries, and Early Salt Distribution | The authors interpret deep basement structural highs in Gulf of Mexico as hotspot tracks. In this interpretation, the basin began to form as the Yucatan experienced continental crustal extension and 22 degrees of counterclockwise rotation (160–150 Ma). This was followed by a further 20 degrees of counterclockwise rotation and seafloor spreading in the gulf. |
Cook (1984) |
Geophysical Anomalies Along Strike of the Southern Appalachian Piedmont | Documents trends in both Bouguer gravity and magnetic anomalies associated with the Appalachians in Georgia and Virginia. |
Crough (1981) |
Mesozoic Hotspot Epeirogeny in Eastern North America | Attributes a 600 km (373 mi.) wide zone of epeirogeny in SE Canada and New England during the Cretaceous and early Tertiary to the Great Meteor hotspot, as evidenced by apatite fission-track dating. |
Daniels et al. (1983) |
Distribution of Subsurface Lower Mesozoic Rocks in the Southeastern United States, as Interpreted from Regional Aeromagnetic and Gravity Maps | Concludes that Brunswick magnetic anomaly must be older than the Mesozoic features that it can be traced over, and is therefore not sourced by South Georgia rift. The authors performed a paleostress analysis of the New England–Quebec igneous province, which provides an alternative interpretation for the distribution of Cretaceous plutons. Dikes display ESE-WNW and ENE-WSW trends and are spatially distributed in three E-W-striking dike swarms 75 by 300 km (47 by 186 mi.) in area. Leucocratic dikes occur closer to plutons and disappear within 3–4km(2–2.5 mi.), likely recording local stress effects due to pluton emplacement. Lamprophyre dikes occur independently of plutons and strike parallel to regional dike swarms, recording regional far-field stresses. Normal faults in the regions display two orientations: 1. E-W-striking normal faults found predominantly in Montreal area are parallel to graben boundaries and axis of the Monteregian Hills, with vertical offsets ranging between 100 and 430 m (328 and 1,411 ft.). 2. NW-SE to WNW-ESE-striking normal faults are oblique to graben boundaries, with less than 100 m (328 ft.) of vertical offset. NW-SE to WNW-ESE faults are older than E-W-striking faults but exhibit crosscutting relationships, suggesting that some were reactivated during formation of the E-W-striking faults. Some E-W-striking brittle faults and joints are observed in several Cretaceous plutons with similar orientations to dikes that are locally crosscut by these normal faults, suggesting that dike emplacement and faulting are contemporaneous. Conjugate sets of NE-WS dextral and ESE-WNW sinistral strike-slip faults and WNW-SSW reverse faults provide evidence for a compressional stress regime postdating emplacement of the Cretaceous plutons |
Note: Only a portion of the table is shown as an example.
Table 4.1.3-1
Criteria Used to Define the Seismotectonic Zones and Mmax Zones
| Criteria Used for Defining Source Zone 2 | |||||
|---|---|---|---|---|---|
Zone |
Earthquake Recurrence Rate |
Mmax |
Future Earthquake Characteristics |
||
Style of Faulting |
Rupture Orientation |
Seismogenic Depth |
|||
| Seismotectonic Zones | |||||
| Atlantic Highly Extended Crust (AHEX) | X |
X |
|||
| Extended Continental Crust–Atlantic Margin (ECC-AM) | X |
X |
|||
| Extended Continental Crust–Gulf Coast (ECC-GC) | X |
X |
X |
||
| Gulf Coast Highly Extended Crust (GHEX) | X |
||||
| Great Meteor Hotspot (GMH) | X |
X |
X |
X |
X |
| Illinois Basin Extended Basement (IBEB) | X |
X |
X |
||
| Midcontinent-Craton (MidC-A, B, C, D) | X |
X |
|||
| Northern Appalachian (NAP) | X |
X |
X |
||
| Oklahoma Aulacogen (OKA) | X |
X |
|||
| Paleozoic Extended Crust (PEZ-N, PEZ-W) | X |
||||
| Reelfoot Rift (RR, RR-RCG) | X |
X |
X |
X |
|
| St. Lawrence Rift (SLR) | X |
X |
X |
X |
|
| Mmax Zones | |||||
| Mesozoic-and-Younger Extension (MESE) | X |
||||
| Non-Mesozoic-and-Younger Extension (NMESE) | X |
||||
The criteria that have been used to define the seismic source zones are indicated with an “X.” Note that none of the seismic source zones are defined based on the criterion of the probability of activity. However, this criterion was used to define the RLME fault sources.
| Source | Alternatives | Report Section |
|---|---|---|
| Charlevoix | Charlevoix | 6.1.1 |
| Charleston | Charleston-local | 6.1.2 |
| Charleston-narrow | ||
| Charleston-regional | ||
| Cheraw Fault | Cheraw fault | 6.1.3 |
| Cheraw fault-extended | ||
| Meers Fault | Meers fault-Quaternary | 6.1.4 |
| Meers fault-extended | ||
| Oklahoma Aulacogen | ||
| Reelfoot Rift Central Fault System–New Madrid North | New Madrid North-short New Madrid North-extended |
6.1.5 |
| Reelfoot Rift Central Fault System–New Madrid South | New Madrid South: Blytheville fault zone New Madrid South: Bootheel lineament |
|
| Reelfoot Rift Central Fault System–Reelfoot Thrust | Reelfoot thrust-short Reelfoot thrust-extended | |
| Reelfoot Rift–Eastern Rift Margin | Eastern rift margin-north | 6.1.6 |
| Eastern rift margin-south/Crittenden County | ||
| Eastern rift margin-south/river (fault) picks | ||
| Reelfoot Rift–Marianna | Marianna | 6.1.7 |
| Reelfoot Rift–Commerce Fault Zone | Commerce fault zone | 6.1.8 |
| Wabash Valley | Wabash Valley | 6.1.9 |
Table 4.2.4-1
Seismotectonic Zones
| Zone Acronym | Seismotectonic Source Zone |
|---|---|
| AHEX | Atlantic Highly Extended Crust |
| ECC-AM | Extended Continental Crust-Atlantic Margin |
| ECC-GC | Extended Continental Crust-Gulf Coast |
| GHEX | Gulf Coast Highly Extended Crust |
| GMH | Great Meteor Hotspot |
| IBEB | Illinois Basin Extended Basement |
| MidC-A, B, C, D | Midcontinent-Craton (various geometries depending on PEZ and RR geometries) |
| NAP | Northern Appalachian |
| OKA | Oklahoma Aulacogen |
| PEZ-N and PEZ-W | Paleozoic Extended Crust narrow and Paleozoic Extended Crust wide |
| RR and RR-RCG | Reelfoot Rift, Reelfoot Rift with Rough Creek Graben |
| SLR | St. Lawrence Rift, including the Ottawa and Saguenay grabens |
Figure 4.1.1-1
Example logic tree from the PEGASOS project (NAGRA, 2004) showing the assessment of alternative conceptual models on the logic tree. Each node of the logic tree represents an assessment that is uncertain. Alternative branches represent the alternative models or parameter values, and the weights associated with each branch reflect the TI Team’s relative degree of belief that each branch is the correct model or parameter value

Figure 4.1.1-2
Example logic tree from the PVHA-U (SNL, 2008) project showing the treatment of alternative conceptual models in the logic tree
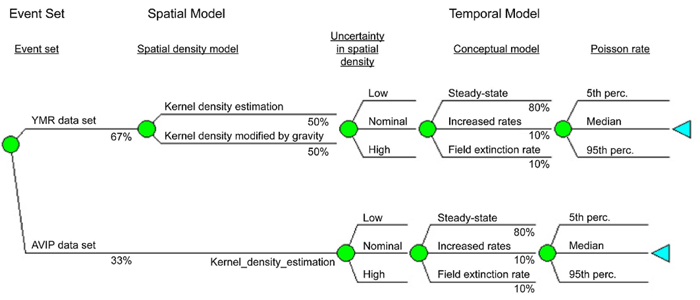
Figure 4.2.1-1
Master logic tree showing the Mmax zones and seismotectonic zones alternative conceptual models for assessing the spatial and temporal characteristics of future earthquake sources in the CEUS

Figure 4.2.2-1
Example of a logic tree for RLME sources. Shown is the tree for the Marianna RLME source
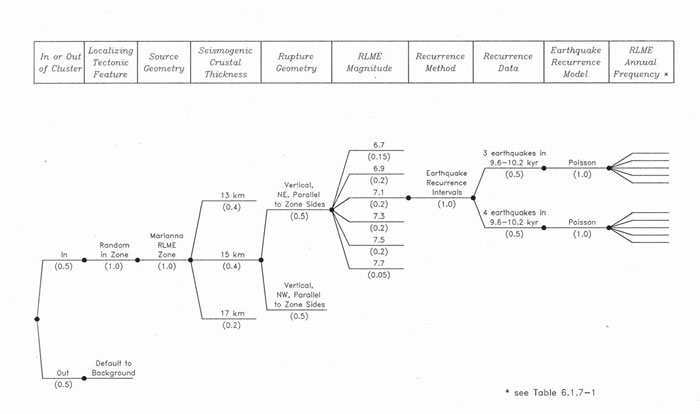
Figure 4.2.2-2
Map showing RLME sources, some with alternative source geometries (discussed in Section 6.1)
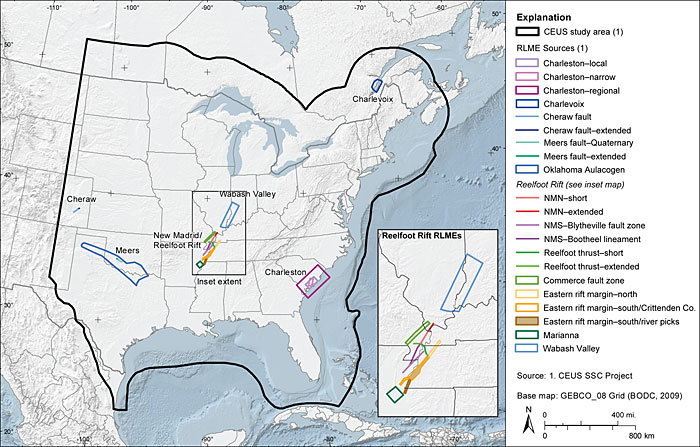
Figure 4.2.3-1
Logic tree for the Mmax zones branch of the master logic tree

Figure 4.2.3-2
Subdivision used in the Mmax zones branch of the master logic tree. Either the region is considered one zone for purposes of Mmax or the region is divided into two zones as shown: a Mesozoic-and-younger extension (MESE) zone and a non-Mesozoic-and-younger zone (NMESE). In this figure the “narrow” MESE zone is shown

Figure 4.2.3-3
Subdivision used in the Mmax zones branch of the master logic tree. Either the region is considered one zone for purposes of Mmax or the region is divided into two zones as shown: a Mesozoic-and-younger extension (MESE) zone and a non-Mesozoic-and-younger zone (NMESE). In this figure the “wide” MESE zone is shown
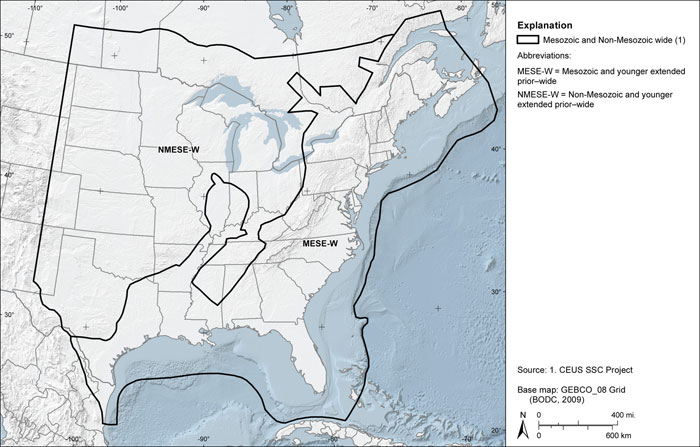
Figure 4.2.4-1(a)
Logic tree for the seismotectonic zones branch of the master logic tree
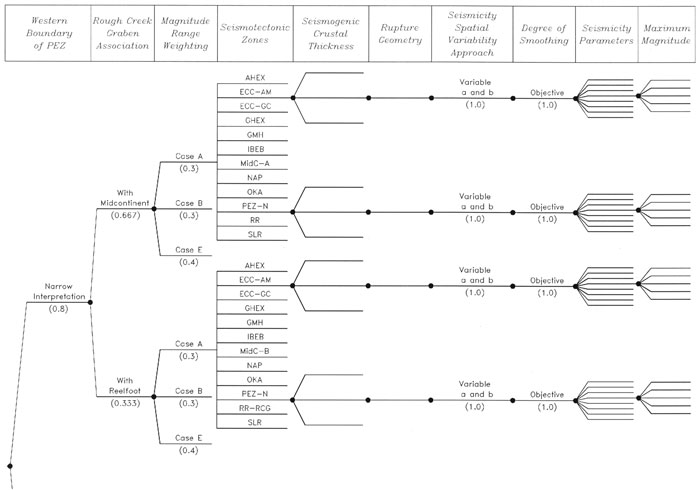
Figure 4.2.4-1(b)
Logic tree for the seismotectonic zones branch of the master logic tree
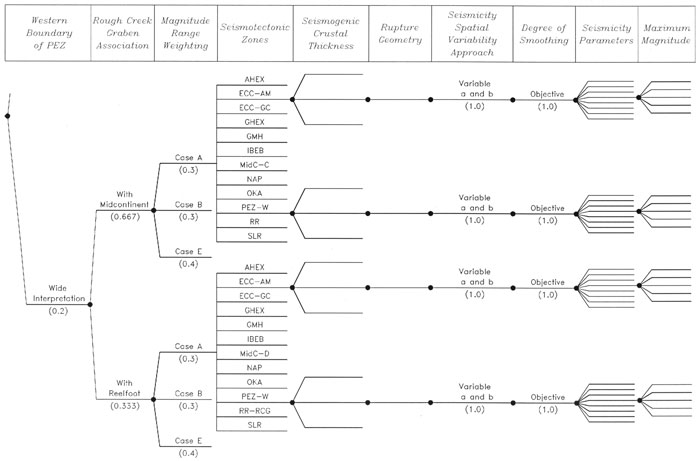
Figure 4.2.4-2
Seismotectonic zones shown in the case where the Rough Creek Graben is not part of the Reelfoot Rift (RR), and the Paleozoic Extended Zone is narrow (PEZ-N)
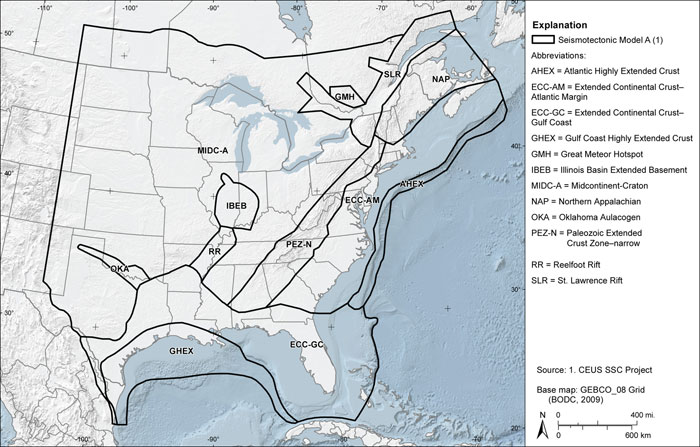
Figure 4.2.4-3
Seismotectonic zones shown in the case where the Rough Creek Graben is part of the Reelfoot Rift (RR-RCG), and the Paleozoic Extended Zone is narrow (PEZ-N).

Figure 4.2.4-4
Seismotectonic zones shown in the case where the Rough Creek Graben is not part of the Reelfoot Rift (RR), and the Paleozoic Extended Crust is wide (PEZ-W)
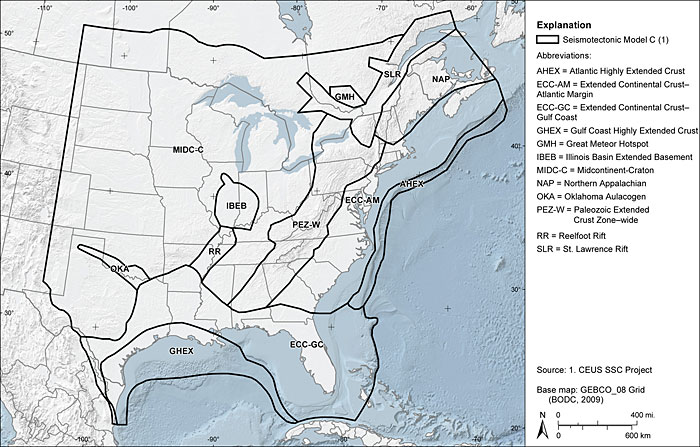
Figure 4.2.4-5
Seismotectonic zones shown in the case where the Rough Creek Graben is part of the Reelfoot Rift (RR-RCG), and the Paleozoic Extended Crust is wide (PEZ-W)